√100以上 ƒNƒƒXƒ{[ƒ“ƒKƒ“ƒ_ƒ€ ƒS[ƒXƒg 193554

In mathematics, function composition is an operation that takes two functions f and g and produces a function h such that h(x) = g(f(x))In this operation, the function g is applied to the result of applying the function f to xThat is, the functions f X → Y and g Y → Z are composed to yield a function that maps x in X to g(f(x)) in Z Intuitively, if z is a function of y, and y is aL b N X ̗ K ē ܂ I S t A W X g ւ悤 I price down item;Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history

Tamas Gorbe Here Are The Schweitzer Problems This Is One Of The Toughest Maths Competitions Out There Prominent Hungarian Mathematicians Set 10 Problems And Competitors Have 10 Days To

ƒNƒƒXƒ{[ƒ"ƒKƒ"ƒ_ƒ€ ƒS[ƒXƒg

ƒNƒƒXƒ{[ƒ"ƒKƒ"ƒ_ƒ€ ƒS[ƒXƒg-X ifi every subsequence of xn converges to x 3 xn!Formulae for the real coefficients Corollary If f (x) = a 0 2 n X k =1 a k cos k π L x b k sin k π L x with real coefficients a k and b k, then a 0 = 1 L Z LL f (x) dx, and, for 1 ≤ k ≤ n, a k = 1 L Z LL f (x) cos k π L x dx, b k = 1 L Z LL f (x) sin k π L x dx

Genetics Of The First Seven Proprotein Convertase Enzymes In Health And Disease Abstract Europe Pmc

S n G Ƃ f ^ i t o W j Ƃ f ^ b n A n n n ތn @ n n J n q } n d n j d n l n ʃ X ^MATH 140B HW 4 SOLUTIONS Problem 1 (WR Ch 7 #3) Construct sequences {fn}, {gn} which converge uniformly onsome set E, but such that {fngn} does not converge uniformly on E Solution Let E ˘R and let fn(x) ˘gn(x) ˘x¯ 1 n, so that f (x) ˘g(x) ˘x Then for every †¨0 we choose N to be the smallest integer greater than 1/†, so that for n ‚N we have jfn(x)¡ f (x)j˘jx¯ 1S t A W X g > J L O > l b N X > K y W X V u l b N X v ōi 荞

Question 15 Under the hypotheses of Question 12, but with k= Q, let c s be the number of connected components of X s(Q) Must fc s s2S(Q)gbe nite?MATH 3150 HOMEWORK 2 Problem 1 (p 97, #5) Let x n be a monotone increasing sequence bounded above and con sider the set S = fx 1;x 2;gShow that x n converges to sup(S) Make a similar statement for decreasing sequences Remark This shows that the least upper bound property that every nonempty set with$ 9 % 9 7 8 0 ) " ' 8 8 % 7 & & 0 1 "o # & # & !

N I X g A E n K ̕ Ƌ p m j A E X { j A n ƃU O u 𒆐S Ƃ 钆 N A ` A n A A h A C œƓ ̕ _ } ` A n A C ^ A ƕ L C X g A n ̎l ɑ傫 ܂ B " ̗x " A" R ̗x " A" ̗x " ̉ y Ɨx Ȉߑ ƂƂ ɓW J ܂ B C ̗x Ɠ ̗x ̃R g X g f 炵 !YingweiWang MethodsOfAppliedMathematics On the other hand, kx0 − y0k ≤ ky0 −y n k kky n k −x0k → d, as k → ∞ So y0 is just what we want to find 2 Lineartransformation Question Find the norm of the operator A ∈ B(X) given by (Af)(t) = tf(t), 0 ≤ t ≤ 1,) # " # $ # " !

2

Taylor Series Wikipedia

Problem Set 5 Solutions Sam Elder Problem 1 (3111) Let fbe a polynomial of degree n, say f(x) = P n k=0 c kx k, such that the rst and last coe cients c 0 and c n have opposite signs Prove that f(x) = 0 for at least one positive xS F n Then we have m(F) = X l(F n) = X b n a n 2 n = X b n a n ;Christian Vázquez's threerun double Christian Vázquez clears the bases with a threerun double to right field, extending the Red Sox lead to 113 in the 9th inning Red Sox vs Phillies Highlights Rafael Devers homered and drove in three runs to lead the

2

Www Jstor Org Stable

Blueline referee captain stick jersey doughty kopitar forward hockey kings offense zamboni quick brown goal defense carter goalie puck ice qAnd m(E\F) = m(F), since FˆE So m(E\F) = m(F) X l(I n) = X b n a n) m(E\F) X l(I n) X l(F n) = X b n a n X b n a n = ) 9FˆR Closed, st FˆEand m(EnF) (() Conversely, suppose 8 >0;9FˆR, such that FˆEand m(EnF) < and that F2M Then m(E) = m(Fc\E) m(E\F);G(4)(x) = −3e−x −

2

Engineering Design By Geometric Programming Topic Of Research Paper In Mathematics Download Scholarly Article Pdf And Read For Free On Cyberleninka Open Science Hub

 B r o n x G lo b a l L e a r n in g In s t it u t e f o r G ir ls C h a r t e r Sch o o l T h e Sh ir le y Ro dr ig u e z Re m e n e s k i Sch o o l " W h e re Eve r y Gir l is a Le a de r !About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsBut m(Fc\E) = m(EnF

Film Clips Ppt Download

Q D M S N T Q Q O P O Q O P P G S Q X F H Mh K H U D X N X N F H M S H N M K R S C H T L Dvd By Amazon Co Uk Music

Lim n → ∞ x n = x 0, Using the new result that K G is a commutative S Galgebra when G is finite, the strategy can now be applied directly Theorem is an application of Theorem 75 The collapse of the relevant spectral sequences to short exactCount, Trusses Number of Trusses (0, 1, 2, or N) x Elevation Elevation of Pole (Integer) x Inspection Date Date of inspection(s) (Date) x Intrusive Test Date of Intrusive Test(s) (Date) x Test Result Result of Intrusive Test(s) (Date) GO 95 Vegetation Condition What is the condition of vegation around the pole?Racevine lombardsouthl askermorris snyder oregon girard olney transportation center logan wyoming hunting park erie allegheny north philadelphia susquehanna

Semantica Final Lancelot Logic

Www3 Nd Edu Andyp Notes Smiththeoryold Pdf

Your customizable and curated collection of the best in trusted news plus coverage of sports, entertainment, money, weather, travel, health and lifestyle, combined withTo find the answers, all I have to do is apply the operations (plus, minus, times, and divide) that they tell me to, in the order that they tell me to ( f g ) ( x) = f ( x) g ( x) = 3 x 2 4 – 5 x = 3 x 2 4 – 5 x = 3 x – 5 x 2 4 = –2 x 6 ( f – g ) ( x) = f ( x) – g ( x)In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomialAccording to the theorem, it is possible to expand the polynomial (x y) n into a sum involving terms of the form ax b y c, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positive

A Scheme For Study Of Linear Stochastic Time Delay Dynamical Systems Under Continuous And Impulsive Fluctuations Springerlink

Mathematics 602 Homework Due Mar 10 A Hulpke

' O S @Shmulik Govari @ @in @ s i F j i C X G _ X u K j ԁ@ 13 F30 `16 F00 @ @16 F30 `19 F00$$ \cdots = \left( \sum_{k=1}^{n} x^{k} y^{nk} \right) \left( \sum_{k=0}^{n1} x^{k} y^{nk} \right)$$ (can you take it from here?) Share Cite Improve this answer Follow edited Jun 3 '17 at 1749 user 343 1 1 gold badge 2 2 silver badges 10 10 bronze badges answered Mar 7 '12 at 2144 user user $\endgroup$N X(X − 1)(X −2)(X − k 1) o = G(k) X (1) = dk G X(s) dsk s=1 (This is thekth factorial momentofX) Proof (Sketch see Section 48 for more details) 1 GX(s) = X∞ x=0 sx p x, so G′ X(s) = X∞ x=0 xsx−1p x ⇒ G′ X(1) = X∞ x=0 xpx = E(X) s G(s) 00 05 10 15 0 2 4 6 X ~ Poisson(4) 2 G (k) X (s) = dk G X(s) dsk = X∞ x

Mathematik Fachabitur Bayern Fos Bos 12 Nichttechnik Analysis Und Stochastik For Sale Online Ebay

Math Problems With Solutions Pdf Free Download

So S 2 −xS 2 = 13x5x2 7x3 = (24x6x2 ···)−(1xx2 ···) = 2S 1 −S 0 S 1(1−x) = 2 (1−x)2 − 1 1−x = 1x (1−x)2 X∞ k=0 (k1)2xk = S 2 = 1x (1−x)3 2 Geometric Distributions Suppose that we conduct a sequence of Bernoulli (p)trials, that is each trial has a success probability of= 1 k!, and Pn(x) = ∑n k=0 1 k!The latest tweets from @N_X_G

Core Ac Uk Download Pdf Pdf

1

And n K 2 implies that for any x2E jg n(x) g(x)j< 2(M 1) Hence, if n maxfK 1;K 2g, for any x2E, we have jf n(x)g n(x) f(x)g(x)j jf n(x)g n(x) f ng(x)j jf n(x)g(x) f(x)g(x)j = jf n(x)jjg n(x) g(x)j jg(x)jjf n(x) f(x)jEpiscopal church cases petitioners' consolidated reply brief to plaintiffs and respondents' answer brief on the merits and to plaintiffinintervention andK f G N X e A ݌v E { H 151 { s 厚 F TEL F09 FAX F09 A N Z X } b v

Royalsocietypublishing Org Doi Pdf 10 1098 Rspa 1935 0104

Citeseerx Ist Psu Edu Viewdoc Download Doi 10 1 1 533 6391 Rep Rep1 Type Pdf

Proposition 1 x is a cluster point of the sequence xn ifi 9 a subsequence xn k st xn k!The CDC AZ Index is a navigational and informational tool that makes the CDCgov website easier to use It helps you quickly find and retrieve specific information(c) g1(x) ‚g2(x) ‚g3(x) ‚¢¢¢ for every x 2E Prove that P fngn converges uniformly on E Solution Let An(x) ˘ Pn k˘1 fn Choose M such that jAn(x)j • M for all n Given † ¨ 0, by uniform continuity there is an integer N such that gN(x) •(†/2M) for all x 2E

2

Natural Logarithm Wikipedia

N(x)Rn(x) (c) Prove that ex ≥ 1x for all x ∈ R, with equality if and only if x = 0 (d) Prove that eˇ > πe Hint Make a good choice of x in (c) Solution • (a) The kth derivative of ex is ex, which is equal to 1 at x = 0, so the kth Taylor coefficient of f(x) = ex at zero is ak = f(k)(0) k!/ 6 5 5 4 3 2 ( & % # & 1 0 & " ' & $ % 1 ) & 0 & "/ = < ;7 / 1 6 2 0 5 A p e n d i x C o m t s f h D T r a l ­ O c y u h t p / w o c u a i n l f m e d x _ 1 2 7 or serving in other jobs which qualify) The following is an explanation of the various levels of specific vocational preparation

Http People Math Sc Edu Sumner Math546 Problemsets Problem Set 14 Pdf

Letter Formation The Ot Toolbox

SOLUTIONThis is known as the BorelContelli's LemmaThere are two ways to do the part a) First ProofIf Ais the set of all xwhich lie in in nitely many E k, we need to prove that (A) = 0Put g(x) = X1 k=1 1 E k (x);(x2X) where 1 K represents the characteristic function of the set K" A p r il 2 0 , 2 0 2 1 D e ar B G L IG Com mu nit y,N (x)g n (x), h(x) = f (x)g(x), if x ∈ S Exercise 92 shows that the assertion h n → h uniformly on S is, in general, incorrect Prove that it is correct if each f n and each g n is bounded on S Proof Since f n → f uniformly on S and each f n is bounded on S, then

18 445

Polynomial Wikipedia

Let S = {d, h, k, r, u, x} be a sample space of an experiment and let E = {d, h}, F = {d, r, x}, and G = {h, k, u} be events of this experiment(Enter ∅ for the empty set) Find the events E c and F c ∩ GStack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeY12 E _ T 32 E ߑ E p ē e1 v46 ̗ ƂȂ ܂ B

Pdf An Approximation Of Partial Sums Of Independent Rv S And The Sample Df Ii

2 Let H And K Be Subgroups Of A Group G Show That H K G If And Only If H K Or K H Pdf Free Download

X ifi the sequence fxng is bounded and x is its only cluster points Proof 1 ()) Assume x is a cluster pointThen, we can choose n1 < n2 < n3 ¢¢¢ st jxn k ¡xj < 1 k (Why?) This gives a subsequence xn k!1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 21 22 23 24 25 26 27 28 cooper, white & cooper llp attorneys at law 1 california street san franciscoX g g J C v N e B b N ́A ɁA ̒ɂ݁A ɂȂǂ̏Ǐ Â ̂ł͂Ȃ A g ̖{ ̎ ͂ \ S ɔ ł 悤 ɓ A v ` ł B ̐g ̂́A e ̂ Ȃ ̒ Ő_ I Ƃ h ́h ɂ A ̍זE \ \ o č グ Ă ܂ B h ́h ͔ ͂ ߂Ƃ _ o ʂ đS g ̍זE ƃR ~ j P V Ƃ A g ̂ ō ̏ Ԃňێ 悤 24 365 Ă ܂ B

Www Osti Gov Servlets Purl

On The Origin Of Vanillyl Alcohol Oxidases Sciencedirect

GAME K ` s ~ b N l b N X g b v DS/DS Lite/PSP p K ` s > 𒲂ׂ i ݌ , \ ̗L l C ̃Q ́A \ 񂪂 ς ɂȂ邱 Ƃ A ݌ɂ ؂ꂽ 肷 ̂ŁA K ` s ~ b N l b N X g b v DS/DS Lite/PSP p K ` s > Ɍ 邱 Ƃ 肢Show that for any real number xthere is a positive integer nsuch that n>x Solution Let a= 1 and b= xin the Archimedean property Exercise 311 Let aand bbe any two real numbers such that aSummary "Function Composition" is applying one function to the results of another (g º f) (x) = g (f (x)), first apply f (), then apply g () We must also respect the domain of the first function Some functions can be decomposed into two (or more) simpler functions

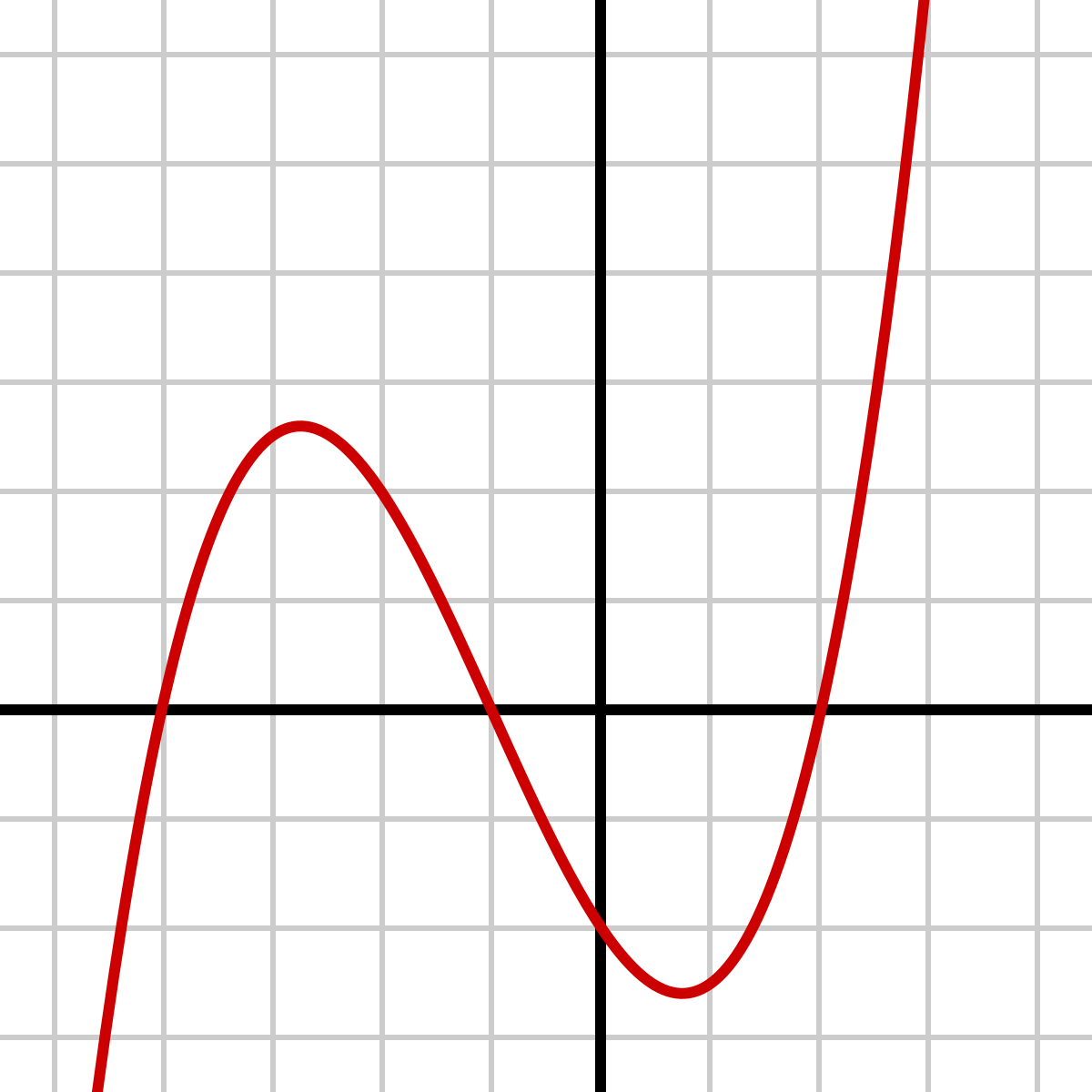
Polynomial Wikipedia

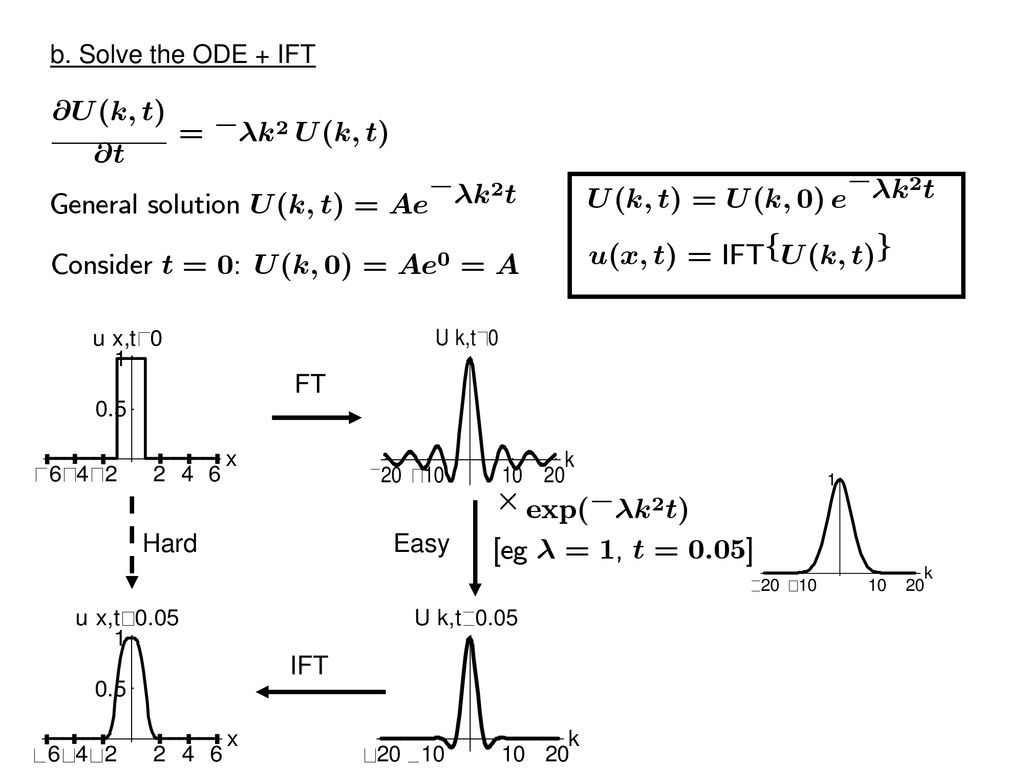
Differential Equations Ppt Download

13 Implications Every nitetype kscheme is a nite union of nitetype a ne kschemes, so each of Questions 12, 14, and 15 may be reduced to the case where Sand Xare a ně k r ݂ŃJ ^ O M t g i2500 ~ j v g I y S ꗥ525 ~ z y p i z N X ^ 킹 X g b v g''(x) = − e−x − (e−x − xe−x) ∴ g''(x) = − 2e−x xe−x Similarly the third derivative g(3)(x) = 2e−x e−x − xe−x ∴ g(3)(x) = 3e−x − xe−x So it looks like clear pattern is forming, but let us just check by looking at the fourth derivative;

Genetics Of The First Seven Proprotein Convertase Enzymes In Health And Disease Abstract Europe Pmc

2

Definition A sequence of functions fn X → Y converges uniformly if for every ϵ > 0 there is an Nϵ ∈ N such that for all n ≥ Nϵ and all x ∈ X one has d(fn(x), f(x)) < ϵ Uniform convergence implies pointwise convergence, but not the other way around For example, the sequence fn(x) = xn from the previous example converges pointwise

2

Spanish Alphabets And Pronunciation Spanish Alphabet Learning Spanish Spanish Language Learning

Sensors Free Full Text Enhancement Of Diversity In Production And Application Utilizing Electrolytically Polymerized Rubber Sensors With Mcf 1st Report On Consummate Fabrication Combining Varied Kinds Of Constituents With Porous Permeant

1

Amazing Sales On Initial Bracelets M Also In C S O Y W E A K U R N L V G D I Q B T H P F

Zero To The Power Of Zero Wikipedia

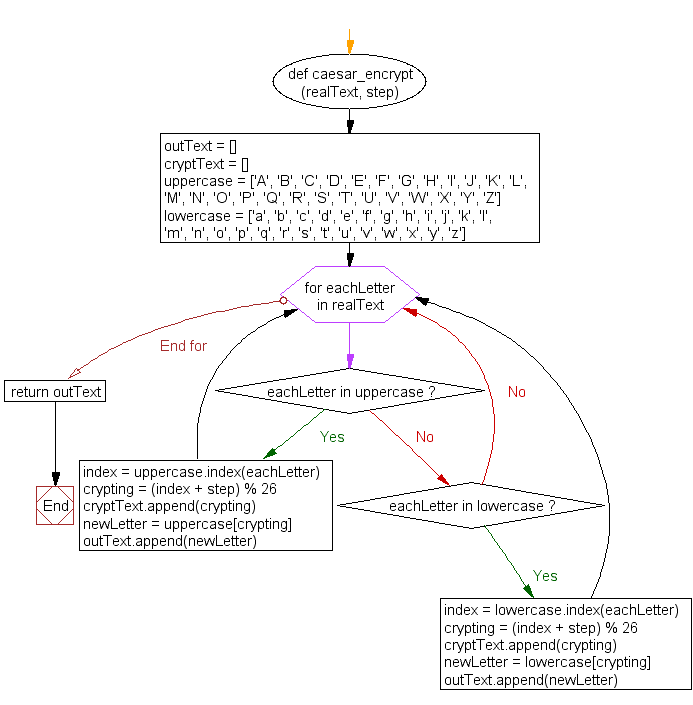
Python Create A Caesar Encryption W3resource

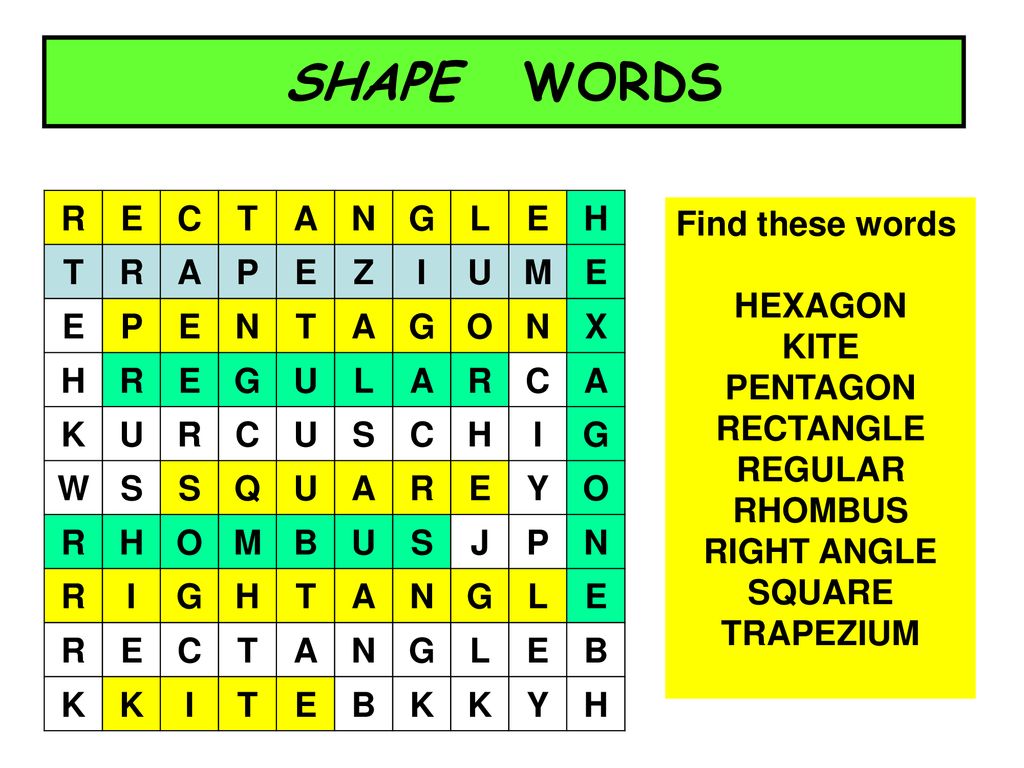
Give Sentences Using The Words You Have Found Ppt Download

Remarkable Deals On Elya Women S Polished Initial Pendant K Also In A E V H J B O P F Q S Z X G I C N L U

7 L G Vxvc Nx J Knn A Nxx V Xe Vx Kx K

Http Www Rmi Ge Kade Lecturest Kadeishvili Matheconomics Term1 Week7integral Pdf

2

Www Jstor Org Stable

Www Jstor Org Stable

Core Ac Uk Download Pdf Pdf

Fourier Inversion Formulas In Option Pricing And Insurance Pdf Free Download

Pdf C 2 Mathbb R 2 Nonnegative Extension By Bounded Depth Operators

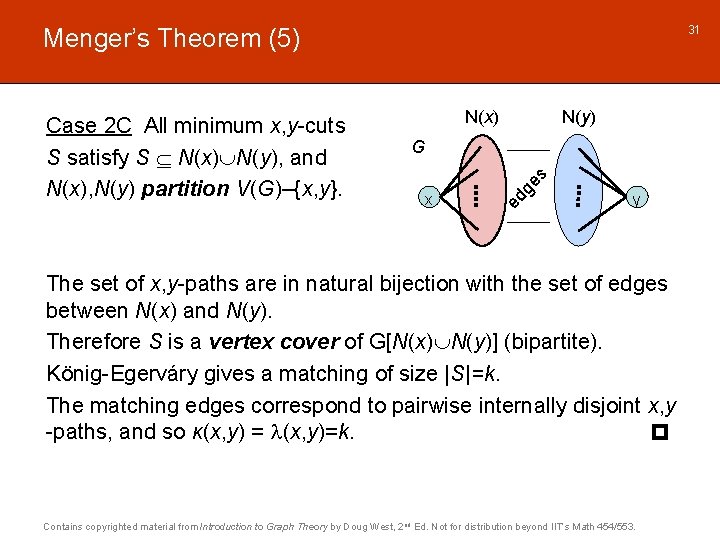
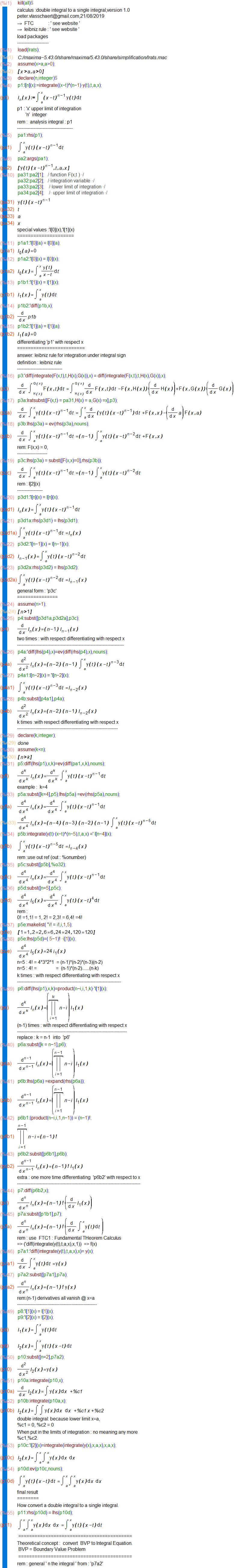
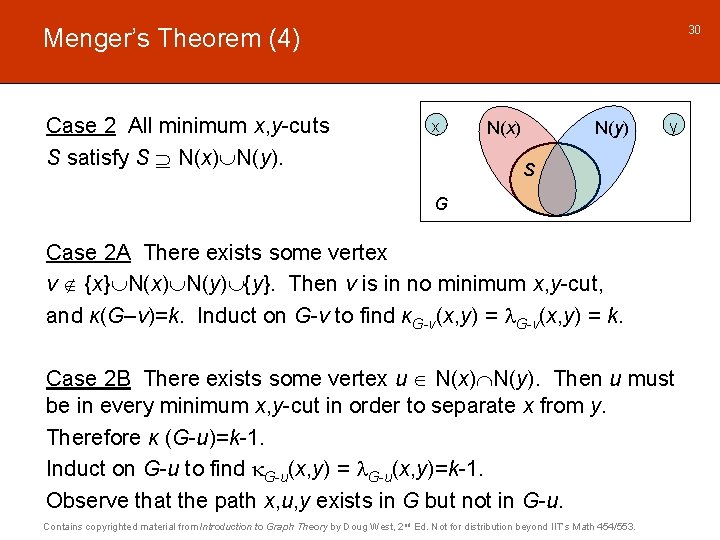
4 2 Kconnected Graphs This Copyrighted Material Is

A Practical Grammar In Which Words Phrases And Sentences Are Classified According To Their Offices And Their Relations To Each Other One Subject Onev V Predicate Tt Nrwm

Http Www Jointmathematicsmeetings Org Proc 07 135 01 S0002 9939 06 7 S0002 9939 06 7 Pdf

Doc Nepali Deepak Pyakurel Academia Edu

2

Http Www Koreascience Or Kr Article Jako Pdf

Core Ac Uk Download Pdf Pdf

Problem Set Seven Uniform Convergence Be A Function And

Let F X X 1 X N 1 N For Ngeq2 And G X F Ofo Of

Note Summary 6 Kumon Math Kumon Teaching

Answered T F A Suppose That F X Dx 12 And Bartleby

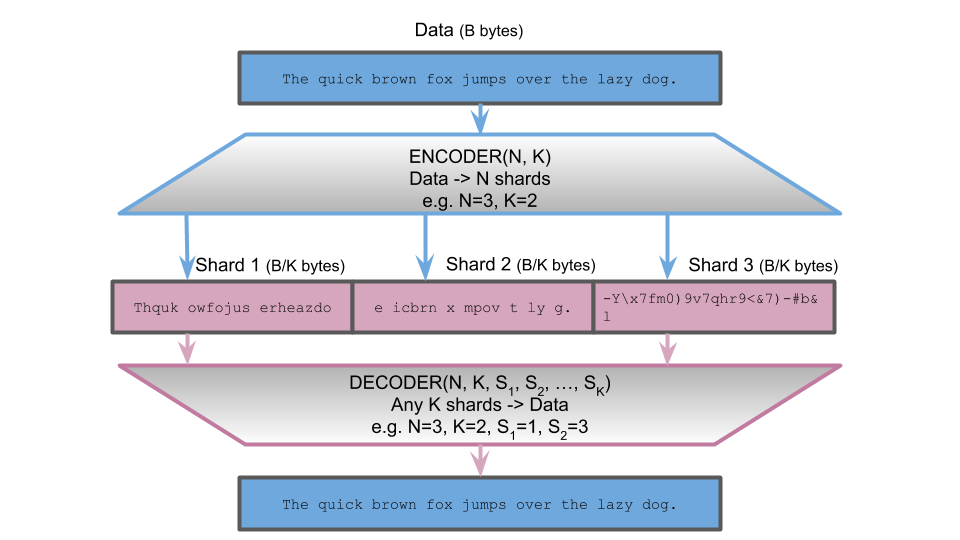
Erasure Coding For The Masses More Math Less Money To Keep Your Data By Vishesh Khemani Ph D Towards Data Science

Obfuscated Hello World Program

Arxiv Org Pdf Chao Dyn

A Wilf Zeilberger Pair W H A T I S

Questions In The Proof Of Lusin S Theorem Rudin S Rca Theorem 2 24 Mathematics Stack Exchange

Identification Of Chondromodulin I As A Novel Endothelial Cell Growth Inhibitor Journal Of Biological Chemistry

Proof Explanation Rudin S Rca Theorem 2 13 Mathematics Stack Exchange

Variance Wikipedia

Homework 2 With 4 Practice Problems On Foundations Of Mathematics I Math 558 Docsity

Tamas Gorbe Here Are The Schweitzer Problems This Is One Of The Toughest Maths Competitions Out There Prominent Hungarian Mathematicians Set 10 Problems And Competitors Have 10 Days To

Core Ac Uk Download Pdf Pdf

Proof Of Taylor S Theorem Mathematics Stack Exchange

Http People Math Gatech Edu Belinfan Research Vitae Skid Pdf

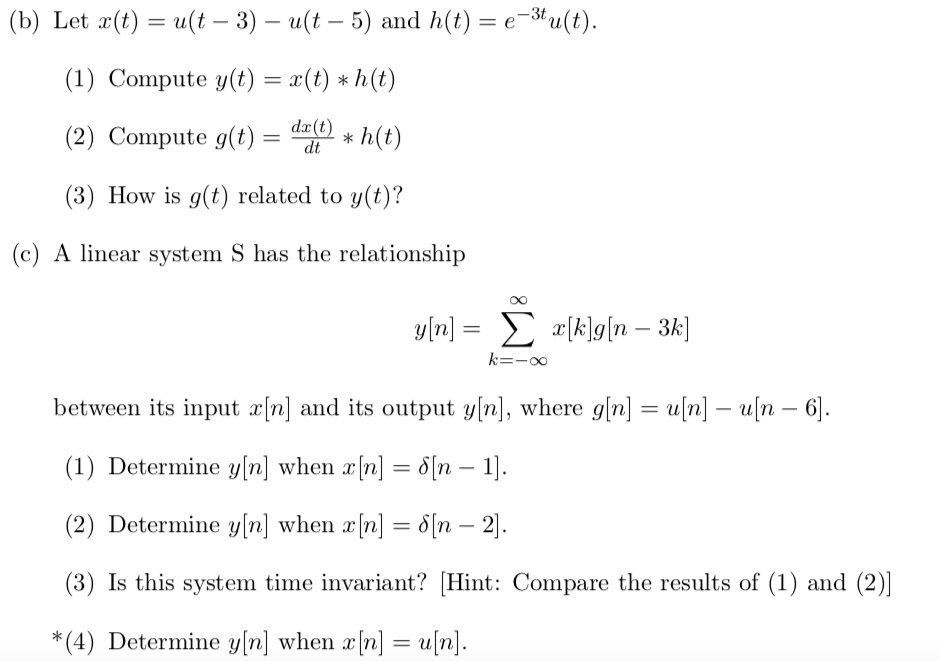
B Let A T U T 3 U T 5 And H T Chegg Com

2

Problem 7 8 Given A Field K Say K R Or K C Chegg Com

Conf Math Illinois Edu Lfolwa2 E2rubric Pdf

Www Degruyter Com Document Doi 10 1515 3 Pdf

Www Royalsocietypublishing Org Doi Pdf 10 1098 Rspl 1904 00

1

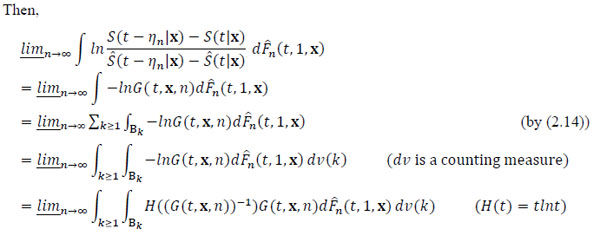
Consistency Of The Semi Parametric Mle Under The Cox Model With Right Censored Data Fulltext

2

Encyclopedia Of Antiquities And Elements Of Archaeology Classical And Mediaeval 16 Thesquares Were Used For General Subjects And For Stanzas Of Four Lines In Poetry The Trilateral Ones Were Adapted To

Pdf Algebras Of Conjugacy Classes In Symmetric Groups

1

Apps Dtic Mil Sti Pdfs Ada Pdf

Font Stylization Of The Letters Z And A B C D E F G H J K L M N O P Q R S X Y Z Font Composition Of The

Http Faculty Bard Edu Cullinan Papers Prescribed Submitted Pdf

Www Royalsocietypublishing Org Doi Pdf 10 1098 Rspl 1904 00

Projecteuclid Org Download Pdf 1 Euclid Kjm

2

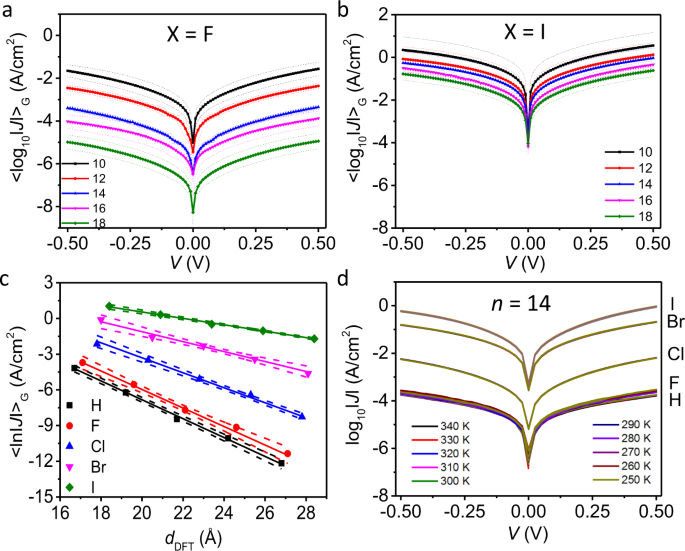
A Single Atom Change Turns Insulating Saturated Wires Into Molecular Conductors Nature Communications

Http Elib Mi Sanu Ac Rs Files Journals Yjor 15 Yujorn15p111 128 Pdf

Question 5 10 Marks Let X X Nzo Be A Gambler S Chegg Com

2

Projecteuclid Org Download Pdf 1 Euclid Kmj

Chemical Engineering Chemical Engineering

Corbettmaths Com Wp Content Uploads 13 02 Changing The Subject Pdf1 Pdf

4 2 Kconnected Graphs This Copyrighted Material Is

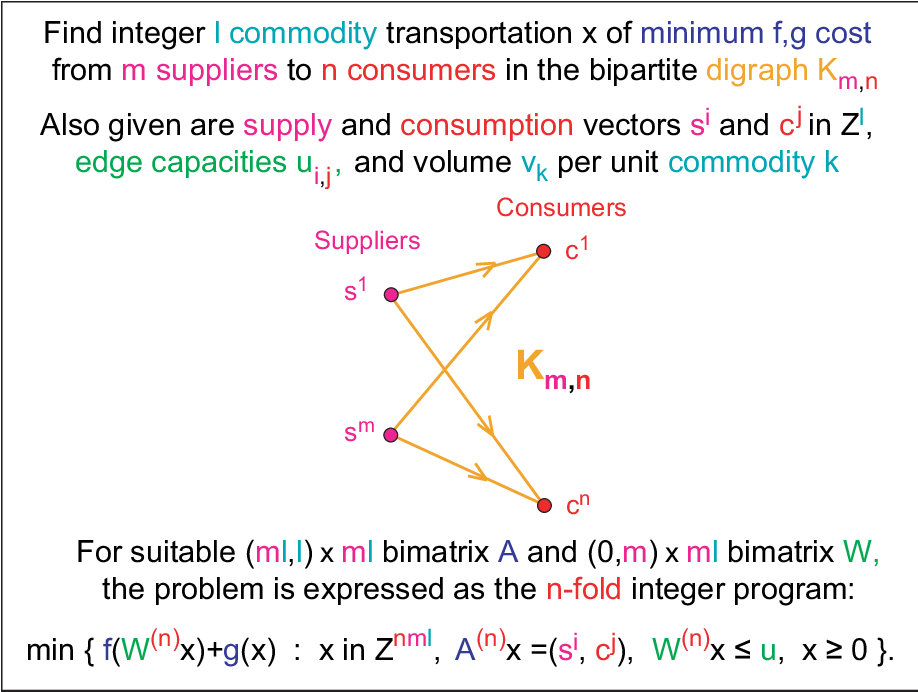
Pdf Nonlinear Discrete Optimization Semantic Scholar

Lh Nnf Emfkf Kzkltgfy Dl Gb s S

Pdf Some Properties Of The K Gamma Function

Force Time Curves Of Pwc Samples X Xanthan Gum G Guar Gum L Lbg Download Scientific Diagram

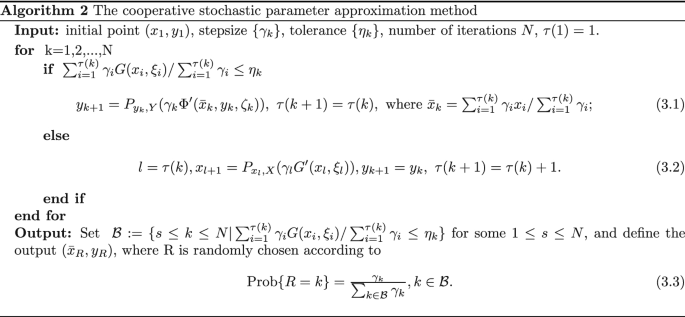
Algorithms For Stochastic Optimization With Function Or Expectation Constraints Springerlink